MEASUREMENT
Measurement
is the process of assigning numbers to observations or events.
In
physics measurement is done to physical quantities, Physical quantities are
categorized as Fundamental physical quantities and derived physical quantities
Fundamental physical quantities (Basic physical
quantities)
These
are physical quantities which cannot be obtained from any simpler physical
quantities. These include Length, Mass, time, temperature, amount of substance,
electric current and luminous intensity.
Derived
physical quantities
Derived
physical quantities are those physical quantities which are obtained (derived)
from other physical quantities. Example velocity, density, force, pressure and
acceleration.
For example.
Velocity
(V)=distance/time thus two basic physical quantities make the velocity which is categorized as derived
physical quantities.
Measurement
of length.
Length
is the distance or displacement between two points.
SI unit
of length is metre (m).
Distance
is the length measured between two points in unspecified direction, for example
20m.
Displacement
is the distance measured between two points in specific direction, for example 20m east. Height and depth are also displacement.
Other
units of distance includes kilometer(km),centimeter(cm),millimeter(mm),micrometer(µm),and
nanometer(nm).
Relationship
between units
1km=1000m
1m=100cm
1cm=10mm
1mm=1000µm
1µm=1000nm
Instruments commonly used to
measure length are
ü
Tape
measure
ü
Vernier
calliper
ü
Ruler
ü
Micrometer
screw gauge
Using tape measure and ruler
Zero
mark of the ruler or tape is placed on the desired initial mark of object to be measured, in some ruler zero
mark is the beginning of the ruler itself while other rulers have zero mark
somewhere beyond the edge, the disadvantage of the former is that edge can be
worn and ruler will give false readings especially when it is not calibrated
into other smaller fractions to use this ruler is safe to measure starting one
then subtract it from the answer
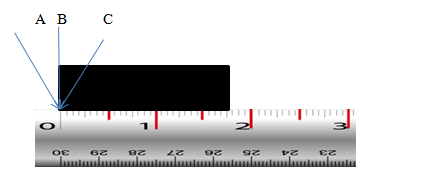
The
ruler above reads 6.5 units, that is the length of object
To read
correctly a ruler one must read at correct position to avoid parallax error
The
correct position to read the ruler is at point B,this avoids parallax error.
Parallax error occurs when a point on an object is viewed from the remaining
two positions, A and B.
VERNIER CALLIPER
A
vernier caliper measures the distance to accuracy of 0.01cm.
Vernier caliper
Parts
of the vernier caliper
·
O-Outside
jaws
·
I-Inside
jaws
·
D-Depth
probe
·
V-Vernier
scale
·
M-Main
scale
·
L-Locking
nut
·
R-Retainer
Typical vernier caliper
diagram
How to read vernier calliper
ü
Read
the main scale
ü
Find
where the vernier scale coincides with the main scale
ü
Take
the position of the coincidence from vernier scale,
ü
Multiply
with 0.01cm
ü
Add
the answer from above to the main scale reading
Example 1.
What
is the reading of vernier caliper below
Solution
Main
scale=6.6cm
Vernier
scale=3 x 0.01cm=0.03cm
Reading
on Main scale + Reading vernier scale
6.6cm+0.03cm=6.63cm.
6.63cm
Example 2.
What
is the reading on vernier below,(units are in cm)
solution
Main scale
reading=1.5cm
Vernier scale=7x0.01cm=0.07cm
Main scale
+vernier scale
=1.5cm+0.07cm
1.57cm
Example 3
What
is the reading on vernier calliper below
Solution
Main
scale reading=10.2cm
Vernier
scale reading=7x0.01cm=0.07cm
Main
scale +vernier scale
10.2cm+0.07cm
10.27cm
MICROMETER SCREW
GAUGE.
Micrometer
screw gauge is used to measure length to the accuracy of 0.01mm(0.001cm).
When
the thimble makes one complete revolution the spindle moves in or out 0.5mm for 50
divisions of thimble or 1mm for 100 divisions of thimble,therefore 0.5mm/50=0.01mm
and 1mm/100=0.01mm
MICROMETER SCREW GAUGE
Parts of micrometer screw gauge
·
A-Anvil
·
C-Circular
scale(thimble scale)
·
F-Frame
·
S-Spindle
·
SL-sleeve
·
R-Ratchet
·
T-Thimble
Diagram of
micrometer screw gauge
Reading micrometer
screw gauge
ü
Read
the sleeve scale
ü
Read
the thimble scale
ü
The
divisions in the thimble scale multiply by 0.01mm
ü
Add
the thimble reading to sleeve reading
Example
1
What is the
reading on the micrometer screw gauge below
Solution
Sleeve reading
=7.5mm
Thimble reading =
22 x 0.01mm = 0.22 mm
Sleeve reading +
thimble reading = 7.5mm + 0.22 = 7.72mm
7.72mm
Example
2
What is the
reading on the micrometer below
Solution
Sleeve reading =
5.5mm
Thimble reading =
30 x 0.01mm = 0.30mm
Sleeve reading +
thimble reading = 5.5mm + 0.30mm
5.80mm
Example
3
What is the
reading on the following micrometer
Solution
Sleeve scale =
14.5mm
Thimble reading =
29 x 0.01mm
Sleeve reading +
thimble reading = 14.5mm + 0.29mm
14.79mm
MEASUREMENT OF VOLUME
Volume
is the space occupied by a body.
Volume of regular shapes can be found by mathematical calculation, Example of
regular shapes includes
cylinder,rectangular,sphere,pyramid and cone
Cylinder
Sphere
cone
Example
Find the volume of a
cylindrical tank of diameter 2m and height 20m.
Solution
V=πr²h
=3.14 x 1²x20
=62.8m³
Volume
of irregular object
Volume of irregular
objects can be obtained by using measuring cylinder as follows
ü
Fill
the measuring cylinder with water and read the initial reading, reading should
be taken at lower part of the meniscus.
ü
Tie
an object with a thin thread
ü
Lower
the object slowly by thread in the cylinder until it is completely immersed in
water.
ü
Read
the final volume of water
ü
Take
the difference between the initial volume and the final volume
ü
The
difference is the volume of irregular object.
What is the volume of
irregular object above?
Solution
Initial reading = 32ml
Final reading = 42ml
Volume=final reading –
initial reading
=42ml-32ml=10ml
Relationship
between units
1m³ =1000litres
1litre = 1000cm³
1ml = 1cm³
1dm³ = 1litre.
MEASUREMENT OF MASS
Mass
is the quantity of matter contained in a body. The SI unit of mass is kilogram(kg).
other commonly used units are ton and gram, ton (t) is mainly used in
industries and gram in laboratory where small amounts of specimen are tested.
Relationship between
units
1 kilogram = 1000grams
1ton = 1000 kilograms
Mass of an object does not change depending on
place it is constant anywhere even on other planet.
The instruments used to
measure mass include:
ü
Beam
balance
ü
Electronic
balance
ü
Triple
beam balance
ü
Lever
balance
·
F
is fulcrum
·
M
is standard mass
·
O
is object
·
P
is pointer
·
S
is scale.
Measurement of mass by
using beam balances is done by comparing (balancing) unknown mass against the
standard mass.
The standard mass is
known mass.
How to use beam balance
1.
Make
sure the pointer points at the middle of scale when nothing is added.
2.
Put
the unknown mass on a scale pan, pointer will shift
3.
Put
the standard mass on another scale pan
4.
Add
the standard masses until the pointer comes to the middle
5.
The
total mass of standard masses is the mass of object (unknown mass).
WEIGTH
The
weight of an object is the force of attraction of the earth on an object
towards its Centre.
Or
The
weight of an object is the force an object exerts on anything which is freely
supporting it.
The SI unit of weight
is Newton (N)
The earth attracts
objects towards its Centre by the pull of gravity.
The value of pull of
gravity on earth’s surface is 9.8N/kg or approximately 10N/kg
Weight = mass x gravity
pull
W=mg.
Weight is measured by
using spring balance.
Spring balance
Parts of spring balance
ü
C-
calibration
ü
S-
spring
ü
P-pointer
ü
h-hook
Example
1
Find the weight of the
mass of 30kg.
Solution
Weight=mass x gravity
W=30kg x 9.8N/kg
W=294N.
294N
Example
2
What is the weight of
an object of mass 50kg on the planet X where the gravity pull is one third of
that of the earth?. What is the difference of the weight of an object on mass
and on planet x?.
Solution
Mass of object=50kg
Gravity pull on earth=
9.8N/kg
Gravity pull on planet
X =1/3 x 9.8N/kg
Weight on planet x =
mass x pull of gravity on planet x
W=50 x1/3 x 9.8N/kg
=163N.
difference between the weight of an object on
the earth and on planet x
weight on earth
=50kg x9.3N/kg
=465N
Difference = 465N-163N
=302N.
Differences
between mass and weight
· mass
is the quantity of matter contained in a body while weight is the force of
attraction of the earth on an object
towards its Centre
·
the
SI unit of mass is kilogram while SI unit of weight is Newton
·
mass
does not change while weight changes
·
mass
is fundamental physical quantity while weight is derived physical quantity
·
Mass
is scalar quantity while weight is vector quantity.
·
mass
is measured by beam balance while weight is measured by spring balance.











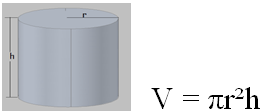





Seriously,these really helps me.
ReplyDeleteIt look much better, than others.
ReplyDeleteThis comment has been removed by the author.
ReplyDeletethese is great
DeleteThis is the best educational site I've been to
ReplyDeleteWow
ReplyDeletethese is great
ReplyDeleteIt is good
ReplyDeleteThanks it's really helping my daughter
ReplyDeleteBien
ReplyDeleteImpressive!
ReplyDeleteI love it
ReplyDelete