A plastic cup containing air (literally empty) when immersed in water upside down it will tend to come up, likewise the piece of wood or bee wax will come up if totally or partially immersed in water
Upthrust force is also known as buoyant force
Buoyant force is an upward force exerted by a fluid to a body which is partially or totally immersed in it and it is equal in magnitude to the weight of fluid displaced by a body.
ARCHIMEDES’ PRINCIPLE (LAW OF BUOYANCY)
States that, when a body is partially or totally immersed in fluid, it experiences an upthrust force which is equal to the weight of fluid displaced.
Experiment to show upthrust is equal to the weight of the fluid displaced
A is a real weight (weight of an object in air) 4.5 N
B is apparent weight (weight of an object in fluid) 3.0 N
C is apparent loss of weight (weight of fluid displaced) 1.5 N
Measuring the weight of displaced fluid is equal to apparent loss of weight; this apparent loss is the buoyancy force on an object which is upthrust.
Conclusion
Apparent loss of weight is equal to the weight of displaced fluid.
Real weight = apparent weight + apparent loss
Since apparent loss is equal to upthrust
Real weight = apparent weight + upthrust
Upthrust =real weight – apparent weight.
Example 1.
A body weighs 5.5 N when measured in air and 3 N when completely immersed in kerosene, what is upthrust experienced by a body.
Solution
Weight of body in air (Real weight) =5.5 N
Apparent weight of a body when immersed in kerosene = 3 N.
But upthrust = real weight – apparent weight loss
Upthrust = 5.5 N – 3 N
= 2.5 N.
Example 2.
A body weighs 3 N when totally immersed in water contained in an overflow can, if weight of displaced water was 2.6 N, what is the real weight of an object.
Solution
Apparent weight = 3 N
Upthrust = 2.6 N (according to Archimedes’ principle)
But
Upthrust = real weight – apparent weight
2.6 N = Real weight – 3 N.
Real weight = 2.6 N + 3 N
= 5.6 N.
DETERMINATION OF RELATIVE DENSITY OF SOLID BY ARCHIMEDES’ PRINCIPLE
If mass is multiplied by gravitational acceleration ‘g’ becomes weight (times 10 above and below which does not change magnitude but changes the physical quantity from mass to weight)
Weight of a given volume of substance: - is the weight of substance in air.
Example 1.
A metal block weighs 108 g in air and 68 g in water, find the relative density of metal. What possibly this metal can be? (Use the table of densities).
Example 2
A body weighs 135 g in air displaced 54 g of water when completely immersed, find the relative density and volume of a body.
Volume of a body is equal to the volume of displaced water.
Density= mass/volume
Therefore 1 g/cm 3= 54 g/volume
Volume = 54 cm 3.
DETERMINATION OF RELATIVE DENSITY OF OBJECT THAT FLOATS BY ARCHIMEDES’ PRINCIPLE
CLASS WORK
AIM: To determine the relative density of an object that floats by Archimedes’ principle.
REQUIREMENTS: floating object, sinker (piece of metal or stone), spring balance, string, and water in a beaker
PROCEDURES:
1. Tie a sinker with a string.
2. Immerse a sinker totally in water and measure its weight (apparent weight) W 1
3. Tie a floating object just before the sinker and immerse totally the sinker leaving the floating object in air then measure weight W 2 (weight of floating object in air + apparent weight of a sinker)
4. Immerse totally both the sinker and the object in water and measure the weight W 3
Example 1
In an Experiment to find the relative density of floating object the followings were observed,
I. Weight of a sinker in air = 64 g
II. Weight of a sinker when totally immersed in water = 49 g
III. Weight of a sinker totally immersed and floating object in air =56 g
IV. Weight when both sinker and floating object totally immersed in water = 46 g
DETERMINATION OF RELATIVE DENSITY OF LIQUIDS BY ARCHIMEDES’ PRINCIPLE
If an object of given volume is totally immersed in different liquids it will always displace volume of given liquid equals to its own volume but the masses of these equal volumes of liquids displaced will differ owing to their densities(concentration of particles)
We can simply know mass of liquid displaced by noticing the apparent loss of mass of an object when totally immersed in liquid; this is according to Archimedes’ principle.
Remember, when mass is multiplied by ‘g’ (gravitational acceleration) it becomes weight also:-
CLASS WORK
AIM: TO FIND RELATIVE DENSITY OF ‘X’
REQUIREMENTS: Liquid x in a beaker, water in a beaker, object that can sink, and spring balance.
PROCEDURES:
1. Measure the sinking object in air and record its weight W 1
2. Measure the sinking object when totally immersed in X and record its apparent weight W 2
3. Measure the sinking object when totally immersed in water and record its apparent weight W 3










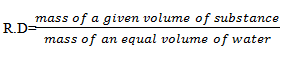

Very fantastic 👍 nice notes
ReplyDeletetheir very helpfull
ReplyDeleteNice work man keep doing
ReplyDeletehttps://gyaanmania.blogspot.com
I like these notes man
ReplyDeleteJust keep it going
Very nice Notes
ReplyDeleteVery nice notes...keep it
ReplyDeleteI love this , keep it up
ReplyDeleteI love it
ReplyDeleteExcellent!!!!!!
I love it
ReplyDeleteExcellent!!!!!!
Good job I love it keep it up sir
ReplyDeleteThis comment has been removed by the author.
ReplyDeleteGood notes, ilike it
ReplyDeleteexcellent work. keep up
ReplyDeleteNice
ReplyDelete